Mathematica package useful for quickly typesetting equations and incorporating derivations into .tex files which can then be included in a main LaTeX document.
For automation related to scientific papers in general, see the auto-paper repository.
The following instructions are adapted from https://mathematica.stackexchange.com/a/672/61736.
- Open
TeXport.min Mathematica - Choose
File-->Install... - Choose
Type-->Package, Source--> (the open notebook),Install Name-->TeXport - Load the package by evaluating (including the
<<):
<<TeXport`Ensure the name is not mispelled (including capitalization).
The Install... menu item will put the package into FileNameJoin[{$UserBaseDirectory, "Applications"}] which on Windows is %AppData%\Mathematica\Applications.
To test that it has installed correctly, open the documentation via:
?TeXportkeys = {a, b, c};
values = {"apples", "bananas", "cantaloupe"};
equation = a + b == c;
keyvalues = {keys, values};
printQ = True;
fname = "texport-example";
TeXport[fname, equation, keyvalues, printQ]
Set printQ to False if you want to export to a file named fname(.tex extension added automatically). Set to True if you want to only Print to $Output (Mathematica's stdout so to speak).
\begin{equation} \label{eq:texport-example}
a+b=c
\end{equation}
where$a$ ,$b$ , and$c$ represent apples, bananas, and cantaloupe, respectively.
If you're new to Mathematica altogether, start with the Documentation Home and look at the linked Working in Notebooks. If you're less familiar with typesetting equations in Mathematica, start by reading this typesetting guide, then in a Mathematica notebook, go to:
Menu Bar --> "Palettes" --> "Basic Math Assistant".
Hover over the various boxes to see the corresponding shortcuts. I recommend using the shortcuts for efficiency, but you can also click on the boxes. If you're interested in the inner-workings of the code, see also String Manipulation Guide. The Mathematica Stack Exchange is another great resource, and many Google searches will direct you to there.
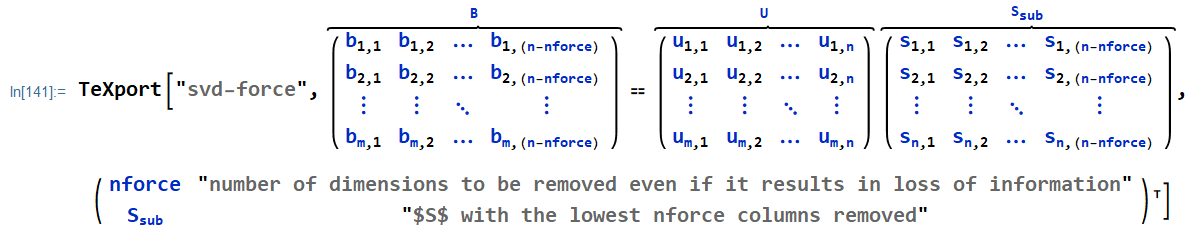
\begin{equation} \label{eq:svd-force}
\overbrace{\left(
\begin{array}{cccc}
b_{1,1} & b_{1,2} & \ldots & b_{1,n-\text{nforce}} \\
b_{2,1} & b_{2,2} & \ldots & b_{2,n-\text{nforce}} \\
\vdots & \vdots & \ddots & \vdots \\
b_{m,1} & b_{m,2} & \ldots & b_{m,n-\text{nforce}} \\
\end{array}gp
\right)}^B=\overbrace{\left(
\begin{array}{cccc}
u_{1,1} & u_{1,2} & \ldots & u_{1,n} \\
u_{2,1} & u_{2,2} & \ldots & u_{2,n} \\
\vdots & \vdots & \ddots & \vdots \\
u_{m,1} & u_{m,2} & \ldots & u_{m,n} \\
\end{array}
\right)}^U \overbrace{\left(
\begin{array}{cccc}
s_{1,1} & s_{1,2} & \ldots & s_{1,n-\text{nforce}} \\
s_{2,1} & s_{2,2} & \ldots & s_{2,n-\text{nforce}} \\
\vdots & \vdots & \ddots & \vdots \\
s_{n,1} & s_{n,2} & \ldots & s_{n,n-\text{nforce}} \\
\end{array}
\right)}^{S_{\text{sub}}}
\end{equation}
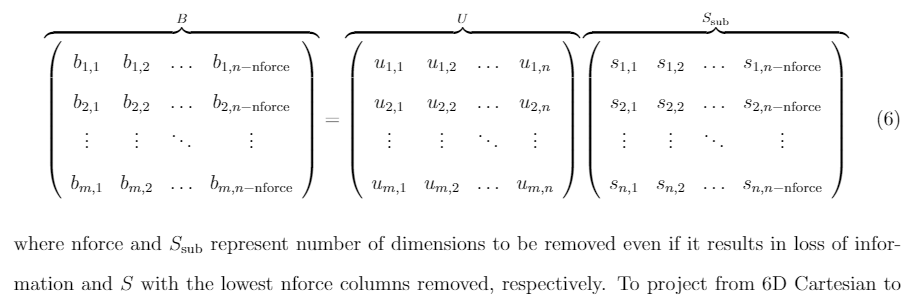
where $\text{nforce}$ and $S_{\text{sub}}$ represent number of dimensions to be removed (even if it results in loss of information) and $S$ with the lowest nforce columns removed, respectively.
You can also perform symbolic computations for a proof and export the entire proof with intermediate commentary as a .tex file. I have done this for a paper I'm working on and will probably put an example together for this. Open up an issue if you'd like to see this sooner.
Once you have your equation .tex files (I suggest putting these in an "equations" folder in your paper repo), commit and push them, and reference the equations in the LaTeX body using e.g. \cref{eq:svd-force} from the cleveref package.