When discussing robot path planning algorithms, there are two basic techniques that are used as a starting point: Probablistic Road Map (PRM), and Rapidly exploring Random Trees (RRT).
Of course there are other techniques, but I enjoy these two methods especially, since they were easy to understand, and personally help me understand graohs a lot better.
My hope with this repository is that the reader also may be able to explore how these algorithms work, and see them in action.
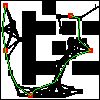
As a prologue, here are the two algorithms in action:
PRM, after it used A* to find the shortest path:
RRT, going from start to end by visiting each waypoints:
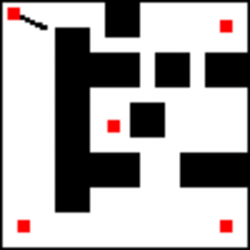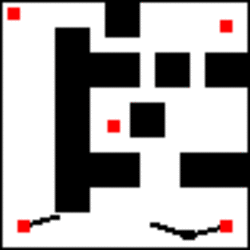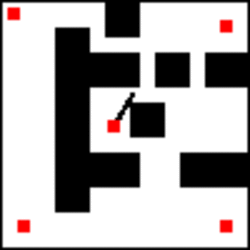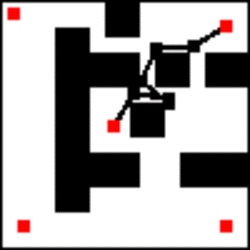
The map, represented by this file. The white spaces represent areas that are available/empty, and black spaces represent areas with obstacle on it. In a real life scenario, this map would based on probability, where each grid represents the probability of it being empty. This is a toy project about path planning, therefore the mapping itself is not the focus.
Probablistic Road Map randomly samples a point, and marks it as a valid vertex if that grid is empty. If that space is occupied with obstacle, then another point is sampled.
After n samples are collected, each vertex finds nearest neighbours that does not have a collision between them. Once the neighbours of each vertices are identified, a graph is formed by connecting these points.
Then, the shortest distance is identified for traversal.
Please check this lecture note from Columbia university for more details.
Rapidly Expanding Random Trees work by expanding a tree from starting point and end point, and joining them once they are close enough. The tree expands py randomly sampling a point, and expanding the graph towards that random point, by taking a step for a give size. The graph is connected once it is confirmed that there is no obstacle present between the graph, and new point.
Please check this lecture note from CMU for more details.